Subsystem symmetry enabling quantum computation
February 26, 2020 - Austin Daniel
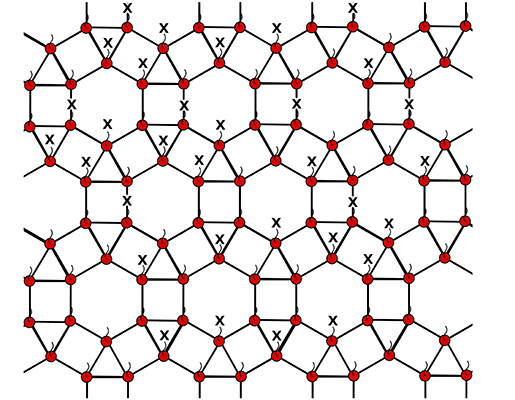Austin Daniel, Rafael Alexander, and Akimasa Miyake have published a paper in the journal Quantum demonstrating that certain many-body quantum systems, when placed on lattices with different geometries, can possess a variety of exotic “subsystem symmetries” that can be utilized to empower quantum computation.
It has long been an open problem to understand which quantum many-body states are useful for measurement-based quantum computing (MBQC), a scheme whereby local measurements on a large, entangled quantum state drive a computational process.
In the past, it was suggested that such systems should possess special topological properties with respect to a symmetry, known as symmetry protected topological order (SPTO).
Traditionally, these many-body systems were studied in terms of a global symmetry that acts uniformly on every site in the lattice; however, recent work has highlighted the importance of subsystem symmetries, which act on special subsets of the lattice.
The authors show that for a variety of lattices there are large families of many-body systems that posses a common subsystem SPTO, ensuring that any such system is a resource for MBQC.
This demonstrates that resources for MBQC extend far beyond what was previously known.
The full article can be found online at https://quantum-journal.org/papers/q-2020-02-10-228/.
