Research

Research Overview

Our Research Group specializes in theoretical quantum information science (QIS), quantum optics, and atomic-molecular-optical (AMO) physics. We study the foundations of quantum information processing (QIP) and its implementation in AMO systems. Key questions we seek to address include:

• How much complexity can we harness for QIP in noisy quantum systems without error correction (NISQ devices)?
• How does dynamical complexity (quantum chaos) relate to computational complexity for QIP?
• How do we achieve high fidelity control of complex quantum systems?
• How do we best characterize, verify, and validate the performance of quantum information processors?

We collaborate with investigators across CQuIC in order to bring fundamental ideas and protocols into the laboratory. Together with Prof. P. S. Jessen, we have developed platforms for quantum control, simulation, measurement, feedback, and tomography, based on spins in cold atomic gases. Together with Profs. T. Albash and A. Miyake we study how much quantum advantage we can harness in Noisy Intermediate Scale Quantum (NISQ) devices. With Prof. G. Biedermann (University of Oklahoma) and Prof. Albash, we seek to put this to the test in an experimental platform based on a Cs Rydberg atom QIP. Together with Prof. F. E. Becerra we seek to create complex quantum states of atom ensembles through measurement and feedback. Together with Dr. M. Martin (Los Alamos) we study quantum control of atomic quditsin an experimental platform basedon a Sr Rydberg atom QIP.

Ongoing Research Projects

Foundational Theory of Quantum Tomography

Use of prior information for robust and efficient quantum state tomography (Joint with P.S. Jessen and C. M. Caves)

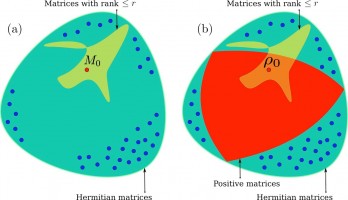A critical task in QIS is the characterization, validation, and verification of the components of quantum information processing devices. Quantum tomography is a standard protocol for achieving this, but the resources required are generally expensive. We study methods to make quantum tomography more efficient without sacrificing its accuracy and precision. This is mainly accomplished by incorporating prior information about the system into the tomographic protocol. For example, most protocols for QIP employ pure quantum states, not arbitrary mixed states. By incorporating such knowledge into our estimators, we can formulate more efficient and robust quantum state tomography (QST) protocols.

- C. H. Baldwin, A. Kalev, and I. H. Deutsch, “Quantum process tomography of unitary and near-unitary maps,” Physical Review A, 90 012110 (2014).

- A. Kalev, R. Kosut, L. and I. H. Deutsch, “Quantum Tomography Protocols with positivity are compressed sensing protocols,” Nature Quantum Information, 1, 15018 (2015).

- C. H. Baldwin, A. Kalev, and I. H. Deutsch, “Informational completeness in bounded-rank quantum state tomography,” arXiv:1510.02735.

- A. Kalev, C. H. Baldwin, and I. H. Deutsch, “The power of being positive: Robust state estimation made possible by quantum mechanics,” arXiv:1511.01433

• Continuous measurement tomography (Joint with P.S. Jessen)

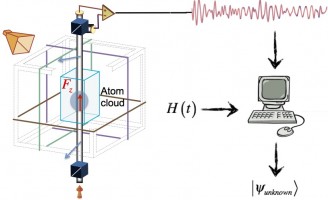A quantum system can be measured continuously by weakly entangling it with a propagating probe (e.g. a laser beam) and then continuously measuring the probe. Such measurements, when applied a many-body ensemble, can be used to perform QST if we simultaneously drive the system with external control fields such that new information is continuously written onto the probe. An informationally complete measurement record is one that specifies the initial quantum state to within a desired accuracy, yielding a fast and robust procedure for QST. This protocol also opens fundamental questions in quantum measurement theory. How is the complexity of the control dynamics related to the information in the measurement record? What is the limit of this protocol, given fundamental tradeoffs between information-gain and disturbance of the state due to measurement backaction. Can one extract many-body correlations between members of the ensemble from the information in the measurement record?

- C. Riofrio, P. S. Jessen, and I. H. Deutsch, “Quantum tomography of the full hyperfine manifold of atomic spins via continuous measurement on an ensemble”, Journal of Physics B15, 154007 (2011).

- V. Madhok, C. A. Riofrío, S. Ghose, and I. H. Deutsch, “Information gain in tomography A quantum signature of chaos,” Physical Review Letters 112, 0414102 (2014).

- R. L. Cook, C. A. Riofrío, and I. H. Deutsch, “Single Shot Quantum State Estimation via a Continuous Measurement in the Strong Backaction Regime,” Physical Review A 90, 032113 (2014).

Quantum Control and Measurement of Qudits

• Optimal control for implementation of arbitrary dynamical maps on qudits (Joint with P.S. Jessen)

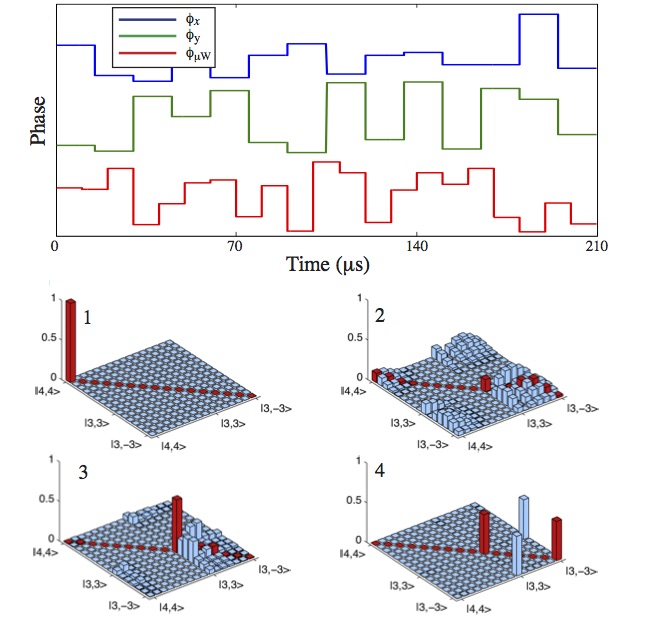We are familiar with “qubits,” the quantum bit, a two-level quantum system that can exist in a superposition of 0 and 1. Qudits are d-level systems, with d>2. Our physical platform is the hyperfine spins of ultracold cesium atoms, which provides a d=16 dimensional qudit Hilbert space. We study methods to control qudits – prepare arbitrary superposition states, embed qubits in qudits, and perform quantum logic gates on qudits. We explore these methods in both and open and closed quantum systems dynamics.

- B. E. Anderson, H. Sosa-Martinez, C. A. Riofrío, I. H. Deutsch, and P. S. Jessen, “Accurate and robust unitary transformation of a high-dimensional quantum system,” Physical Review Letters 114, (2015).

• Implementation of fundamental POVMs for tomography (Joint with P.S. Jessen and F. E. Becerra)

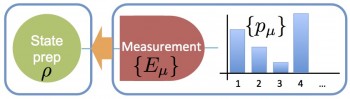Any quantum measurement can be succinctly described by a positive operator-valued measure (POVM), a set of operators that determine the probabilities of the different measurement outcomes, given a quantum state. But, which POVM is best for quantum tomography? The answer to this question depends on the context: efficiency of implementation, robustness to statistical noise, and systematic errors. We explore these questions both theoretically and in real-world laboratory implementations. This applies to three “flavors” of tomography: state tomography, process tomography, and detector tomography.

Collective spin-control

• Spin squeezing of ensembles of qudits (Joint with P.S. Jessen)

The collective spin of an ensemble of atoms constitutes a simple many-body system in which to explore new approaches to quantum control and measurement. A benchmark for such control is “squeezing” of the quantum uncertainty of the collective spin’s direction along some axis, with applications in precision metrology. Spin squeezing arises from entanglement between the constituent spins and is also of fundamental interest in QIP and many-body physics. We study the generation of spin squeezing based on measurement backaction. Since backaction arises from entanglement with a laser probe, we study the use of quantum control of the constituent spin qudits enhance the atom-light interface for improved squeezing.

- L. M. Norris, C. M. Trial, P. S. Jessen, and I. H. Deutsch, “Enhanced Squeezing of a Collective Spin via Control of Its Qudit Subsystems,” Physical Review Letters 109, 173603 (2012).

- B. Q. Baragiola, L. M. Norris, E. Montaño, P. G. Mickelson, P. S. Jessen, and I. H. Deutsch, “Three-dimensional light-matter interface for collective spin squeezing in atomic ensembles,” Physical Review A 89, 033850 (2014), Editors Choice.

• Nanophotonic atom-photon interface(Joint with P.S. Jessen and Y.-Y. Jau)

AMO platforms for QIP require strong coupling between atoms and photons. We explore the use of nanophotonics to engineer an entangling atom-light interface and apply it to new protocols. One example is an optical nanofiber, in which atoms are trapped in the evanescent field of the waveguide to form one-dimensional optical lattices on two opposite sides of the fiber. With a long string (of order of a few thousand atoms) along the length of the nanofiber, one can achieve strong entangling cooperativity between the atomic ensemble and the guided mode. In order to implement a fully functional quantum interface we must address a number of challenges. Amongst these include how to cool the trapped atoms into the motional ground state and prepare the atomic ensemble in an arbitrary quantum state.

- X. Qi, B. Q. Baragiola, P. S. Jessen, and I. H. Deutsch, “Dispersive response of atoms trapped near the surface of an optical nanofiber with applications to quantum demolition measurement and spin squeezing,” Physical Review A, 93, 023817 (2016).

• Rydberg mediated spin-spin interactions (Joint with G. Biedermann and Y.-Y. Jau)

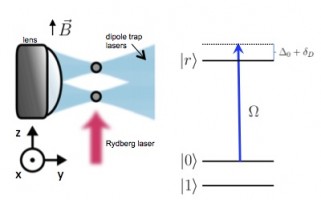Neutral atoms are at the heart of the world's most quantum coherent device – the atomic clock. Fundamentally, this stems from the long coherence times associated with qubits encoded in atomic spins, and our ability to control them with coherent magneto-optical fields. The next frontier is coherently controlling the interactions between atoms to produce entangled states for QIP. A powerful mechanism for doing this is the strong electric dipole-dipole interaction between atoms, excited to high-principle-quantum-number Rydberg states. We study how to employ this mechanism in order to mediate spin-spin entangling interactions for applications in quantum computing, simulations, and metrology.

- T. Keating, R. L. Cook, A. Hankin, Y-Y Jau, G. W. Biedermann, and I. H. Deutsch, “Robust quantum logic in neutral atoms via adiabatic Rydberg dressing,” Physical Review A 91, 012337 (2015).

Y-Y Jau, A. Hankin, T. Keating, I. H. Deutsch, and G. W. Biedermann, “Entangling atomic spins with a Rydberg-dressed spin-flip blockade,” Nature Phys. 12, 71 (2016).

Quantum Computation and Simulation

• Analog vs. Digital Simulation: Computation complexity and robustness to errors

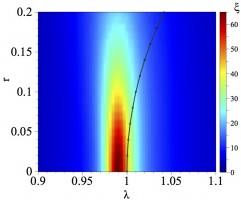One of the great promises of QIP is the ability to simulate quantum mechanics itself. A quantum simulation can be performed on a universal, digital, quantum computer, with appropriate algorithms. An alternative strategy is to employ an analog quantum simulator, whose operation is based on emulating the target Hamiltonian and its continuous-time evolution without resorting to a digital quantum-logic network. The prevailing view is that the requirements on control and mitigation of decoherence are far less demanding for an analog quantum simulator than for a digital quantum computer that requires fault-tolerant quantum error correction. We are working to evaluate this perspective critically and to develop our understanding of the computational power of analog quantum simulation.

- P. Hauke, F. Cucchietti, L. Tagaliacozzo, M. Lewenstein, and I. H. Deutsch, “Can one trust quantum simulators?” Reports on Progress in Physics 75, 082401 (2012).

• Case study: Linear optics Boson sampling; Bose-Hubbard model (Joint with A. Miyake)

Boson sampling is a deceptively simple protocol. Single photons are injected into multiple ports of a linear optical network and photons are counted at the output ports. The number of clicks arising at each output port is random, due to quantum inference between the multiple paths in the interferometer. These random numbers are samples from a probability distribution that is provably hard to simulate on a classical computer (under certain assumptions of computationally complexity). This paradigm provides a launching pad for explorations of the computational power of complex quantum systems. How is sampling complexity connected to the entanglement in the quantum state? How is sampling complexity related to quantum simulations, particularly in interacting many-body system? We use of the Bose-Hubbard model, typically applied to cold atoms in optical lattices, to bridge between the paradigm of boson sampling in linear optics and quantum simulations of condensed-matter phenomena.