Optimal Pure-State Qubit Tomography via Sequential Weak Measurements
October 17, 2018 - Gloria Cordova
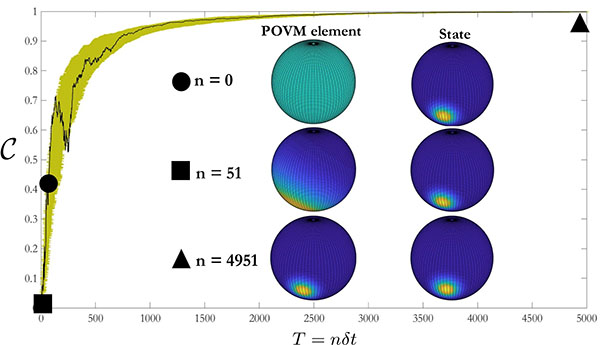
A sequence of weak isotropic measurements can yield the optimal measurement of a collection of identical qubits — a POVM over the continuous set of spin-coherent states. We show here a numerical simulation for an independent sequence of collective isotropic weak measurements on an ensemble of 50 qubits. The vertical axis is a measure how close a POVM element is to a spin-coherent state projector with the value the “coherency” C=1 corresponding to a spin-coherent state. The olive region consists of the coherency for 50 samples of the measurement record as a function of time. The spheres are Husimi distributions of the POVM element (left) and the post-measurement state (right) for various times of the black sample trajectory. The POVM converges asymptotically, extracting the estimated direction of the unknown spin, whilst the state does not change drastically, because the measurements are weak.
It’s been known since the early days of quantum information science that the optimal way to gain information about a quantum state given many copies is to do a joint measurement of the whole ensemble. An example is seen in quantum state tomography. In 1995, Massar and Popescu proved that the optimal measurement for estimating an unknown direction of N copies of a pure qubit is a collective measurement. The realization for every N is the so-called spin-coherent-state POVM. After various attempts, by 2002 many researchers had come to the conclusion that this measurement was not practically realizable for large N. However, in recent work published in Physical Review Letters (give link) researchers at CQuIC showed that an implementation of the spin-coherent-state POVM could indeed be implemented via a sequence of collective and isotropic weak measurements without feedback or adaptivity.
Spin-coherent states are quantum states that transform in the same way as a vector under 3-dimensional rotations.This vector can be a literal direction in 3D real space but can also be an abstract direction such as in the Bloch sphere of a qubit. For N copies of a qubit, these directions are represented by states which sit in an irreducible subspace that spans N+1 of the 2^N dimensions of the total Hilbert space. This subspace is also known as the spin-J=N/2 representation of SU(2). The spin-coherent-state measurement of a spin-J system is a measurement where the outcomes are the continuum of directions which is represented by a POVM with elements that are projectors onto the spin-coherent states. The weak measurement record eventually converges on the estimated direction.This protocol could be implemented on currently existing platforms, such can be done using the Faraday interaction to measure the collective spin projection of an atomic ensemble with a continuous laser probe.
With this new breakthrough, CQuIC graduate student Ezad Shojaee and postdoc Chris Jackson, together with Prof. Ivan Deutsch are working on applications for more generalized coherent-state measurements such as those with SU(1,1) symmetry and other semi-simple Lie group symmetries.
This work was published in the September 26, 2018 issue of Physical Review Letters:
Optimal Pure-State Qubit Tomography via Sequential Weak Measurements
Ezad Shojaee, Christopher S. Jackson, Carlos A. Riofrío, Amir Kalev, and Ivan H. Deutsch
Phys. Rev. Lett. 121, 130404
